Uniform Continuous Distribution Fitting

The continuous uniform distribution or rectangular distribution is a family of symmetric probability distributions such that for each member of the family, all intervals of the same length on the distribution's support are equally probable. The support is defined by the two parameters, a and b, which are its minimum and maximum values. The distribution is often abbreviated U(a,b). It is the maximum entropy probability distribution for a random variate X under no constraint other than that it is contained in the distribution's support.

References:

• Wikipedia, The Free Encyclopedia. Uniform Distribution (continuous). Available on: http://en.wikipedia.org/wiki/Uniform_distribution_(continuous)

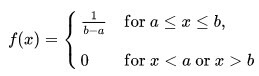
Where:

• a is lower bound

• b is upper bound

We used Accord.Statistics for this calculator

Paste a column data here. In format of excel, text, etc. Each sample in one line.

Calculate the probability of the fitted distribution on: Uniform-Continuous Distribution Calculator

Uniform Continuous Distribution: